Calculators
::
2D Shapes
::
Equilateral Triangle Calculator

Equilateral Triangle Calculator

Input one element of an equilateral triangle, and the calculator will find the five unknown elements. The calculator will provide a step-by-step explanation on how to calculate missing elements.

Equilateral triangle calculator

Input the side, perimeter, area, circumcircle radius or altitude of an equilateral triangle, then choose a missing element.
help ↓↓ examples ↓↓ tutorial ↓↓

Solve for

Input one element of an equilateral triangle.

side

a =

height

h =

perimeter

P =

area

A =

circumcircle radius

R =

incircle radius

r =

thumb_up 218 thumb_down

Get Widget Code

working...

Hire MATHPORTAL experts to do math homework for you.

Prices start at $3 per problem.

Equilateral triangle formulas

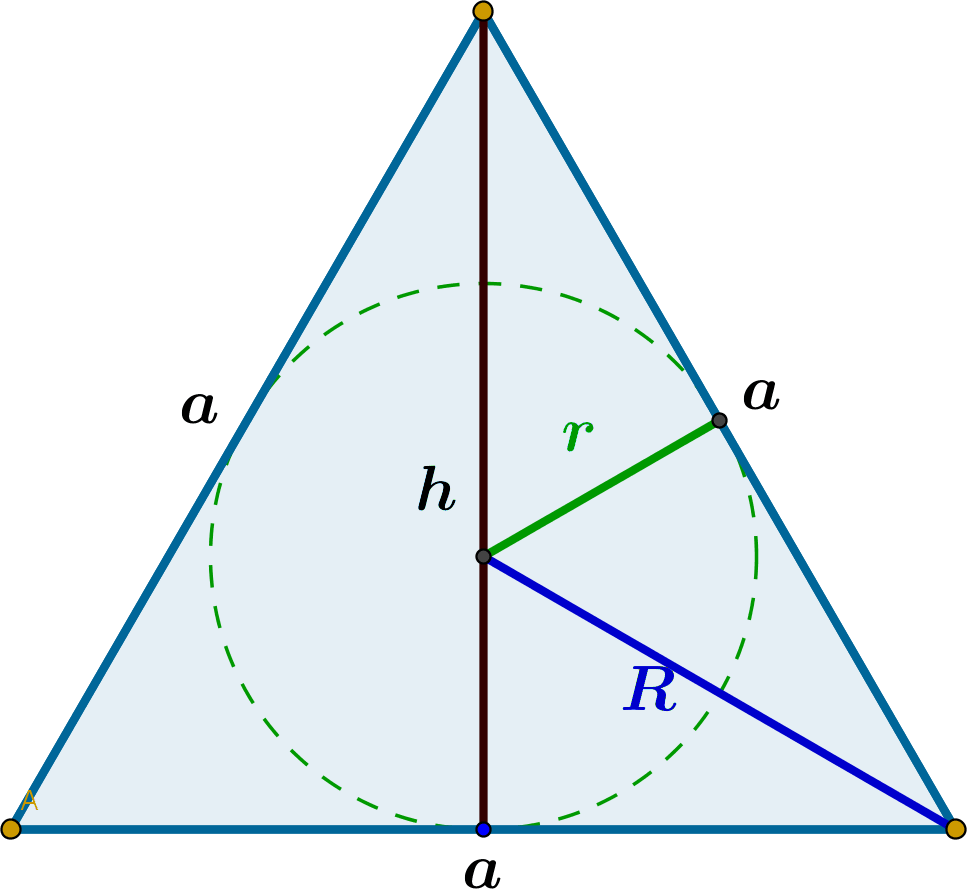
Equilateral triangle with sides, height and incircle radius.

$$ A = \frac{3 \, a^2 \sqrt{3}}{4} $$

area

$$ h = \frac{a \sqrt{3}}{2} $$

height

$$ r = \frac{a \sqrt{3}}{6} $$

incircle radius

$$ R = \frac{a \sqrt{3}}{3} $$

circumcircle radius

Examples

ex 1:

What is the area of an equilateral triangle of perimeter $P = 6\sqrt{2}$.

ex 2:

What is the perimeter of an equilateral triangle if its height is $\dfrac{20}{3} cm^2$?

ex 3:

If base of an equilateral triangle 50 inches long, what is the triangle's height?

ex 4:

$\triangle ABC$ is an equilateral triangle with area A = 24. Find the perimeter.

Related calculators

Right triangle calculator

Find more worked-out examples in our database of solved problems..

TUTORIAL

Equilateral triangle calculations

This calculator uses the following formulas to find the missing values of a triangle.

Perimeter:	$$ P = 3 \cdot a $$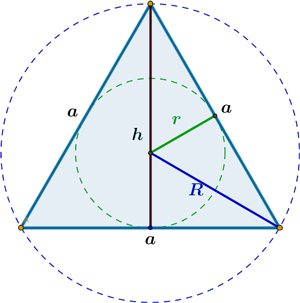
Area:	$$ A = \frac{a^2 \sqrt{3}}{4} $$
Height:	$$ h = \frac{a \sqrt{3}}{2} $$
Circumcircle radius:	$$ R = \frac{a \sqrt{3}}{3} $$
Incircle radius:	$$ r = \frac{a \sqrt{3}}{6} $$

Example 01 :

What is the area of an equilateral triangle whose side is $ 12 cm $.

Solution:

In this example we have $ a = 12 $.

To find the area we will use formula $A = \dfrac{a^2 \sqrt{3}}{4} $

$$ \begin{aligned} A & = \frac{a^2 \sqrt{3}}{4} \\[ 1 em] A & = \frac{12^2 \sqrt{3}}{4} \\[ 1 em] A & = \frac{144 \sqrt{3}}{4} \\[ 1 em] A & = 36 \sqrt{3} \end{aligned} $$

solve this problem using calculator

Example 02 :

What is the side of an equilateral triangle whose height is 15 cm?

Solution:

In this example we have $ h = 15 $.

To find height we will use formula $h = \dfrac{a \sqrt{3}}{2} $

$$ \begin{aligned} h & = \frac{a \sqrt{3}}{2} \\[ 1 em] 15 & = \frac{a \sqrt{3}}{2} \\[ 1 em] a \sqrt{3} & = 15 \cdot 2 \\[ 1 em] a \sqrt{3} & = 30 \\[1 em] a & = \frac{30}{\sqrt{3}} \cdot \frac{\sqrt{3}}{\sqrt{3}} \\[1 em] a & = \frac{30 \sqrt{3}}{3} \\[ 1 em] a & = 10 \sqrt{3} \approx 17.3 \end{aligned} $$

solve the problem using calculator

Search our database with more than 300 calculators

362 861 664 solved problems